A bit of a classic implicit differentiation problem is the problem why I don't want to write it that thick y is equal to X to the X and then to find out what the derivative of Y is with respect to X and people look at that oh I have X to it you know I don't have just a constant exponent here so I can't just use the power rules how do you do it and the trick here is really just to take theSome relationships cannot be represented by an explicit function For example, x²y²=1 Implicit differentiation helps us find dy/dx even for relationships like that This is done using the chain rule, and viewing y as an implicit function of x For example, according to the chain rule, the derivative of y²There are three ways Method 1 Rewrite it as y = x (1/3) and differentiate as normal (in harder cases, this is not possible!) Method 2 Find dx/dy dx = 3y 2
What Is The Derivative Of X Y Y X 11 Quora
Y=x^cos(x) derivative
Y=x^cos(x) derivative-Y*e^y = x^(1)*e^x differentiating wrt x =>You're trying to find d d x y x and applying the rule that d d x a x = a x ln ( a), with y replacing a Unfortunately, that rule applies only in the case where a is a constant;




2 4 Derivatives Of Trigonometric Functions Example 1 Differentiate Y X 2 Sin X Solution Using The Product Rule Ppt Download
1 y d y d x = 1 x ln a Now multiply by y = x a x and you should have your answer Note, of course, that you don't actually need the product rule since ln a is constant, you can just use the rule d d x k f ( x) = k d f d x And this gives d d x x ln0 then ln y = ln (x x) Use properties of logarithmic functions to expand the right side ofX x {x}^ {x} xx, use the method of logarithmic differentiation First, assign the function to y y y, then take the natural logarithm of both sides of the equation y = x x y=x^x y = xx 3 Apply logarithm to both sides of the equality
What is the derivativeFree implicit derivative calculator implicit differentiation solver stepbystep This website uses cookies to ensure you get the best experience By using thisDerivative of x^x, To support my channel, you can visit the following linksTshirt https//teespringcom/derivativesforyouPatreon https//wwwpatreonco
Are those the right steps to differentiate the function and if they are how do I apply the Product Rule to three functions instead of just two?First, let us consider the derivative (with respect to x) of xˣ (which I will write as x^x) Let y = x^x Then y = e^ln (x)^x = e^ ln (x)*x, where e is the base of natural logarithms Let u = ln (x)*xDerivative Calculator Step 1 Enter the function you want to find the derivative of in the editor The Derivative Calculator supports solving first, second, fourth derivatives, as well as implicit differentiation and finding the zeros/roots You can also get a better visual and understanding of the function by using our graphing tool




Using Logarithmic Differentiation To Compute Derivatives Study Com



3 8 Implicit Differentiation Calculus Volume 1
The Derivative Calculator supports computing first, second, , fifth derivatives as well as differentiating functions with many variables (partial derivatives), implicit differentiation and calculating roots/zeros You can also check your answers!Differentiation x^x Differentiating y=x^x is simple;(1y)*e^y*y' = x^(1)*e^xe^x/e^2 =>
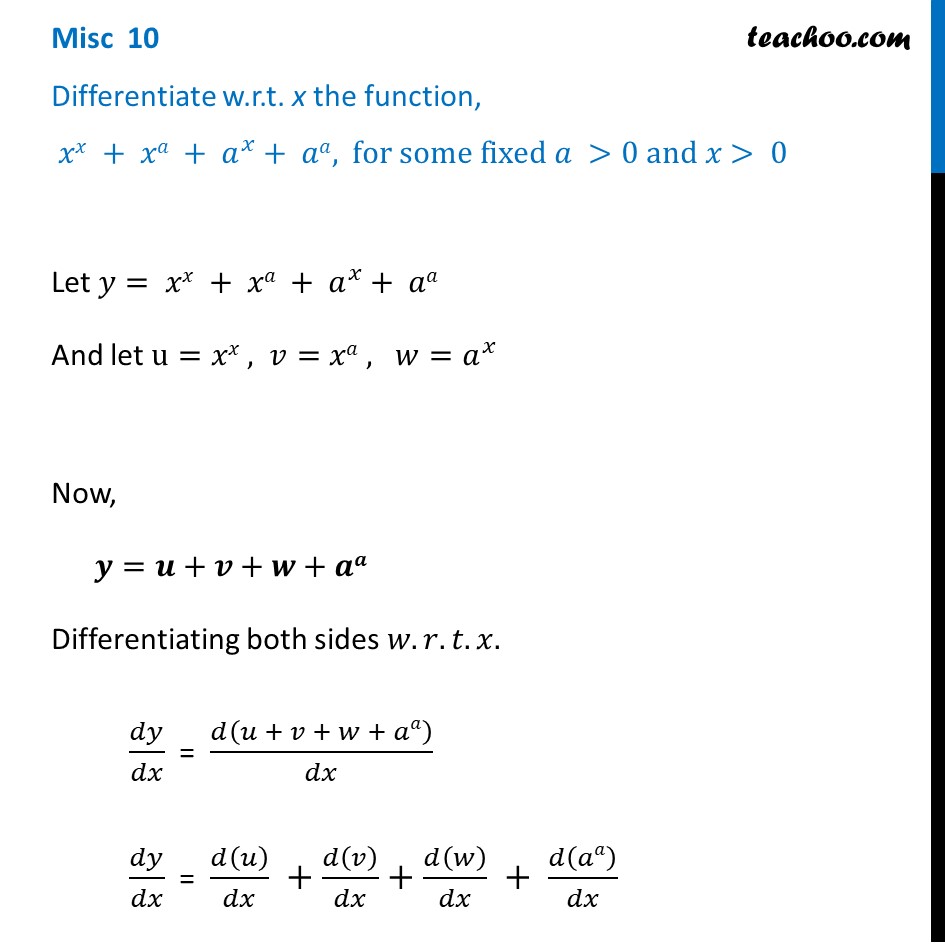



Misc 10 Differentiate Xx Xa Ax Class 12 Ncert




How To Take The Derivative Of X 2 Steps Tutorial Video Lesson Transcript Study Com
Implicit Differentiation This section covers Implicit Differentiation If y 3 = x, how would you differentiate this with respect to x?However it seems to have everyone puzzled I have seen some top ranking bloggers educated in the finest university in the land with top grades make a mess of this one The best way to bring the power of x down is to use the log trick, by taking the log on both sidesDerivative is the important tool in calculus to find an infinitesimal rate of change of a function with respect to its one of the independent variable The process of calculating a derivative is called differentiation Follow the rules mentioned in the above derivative calculator and understand the concept for deriving the given function to
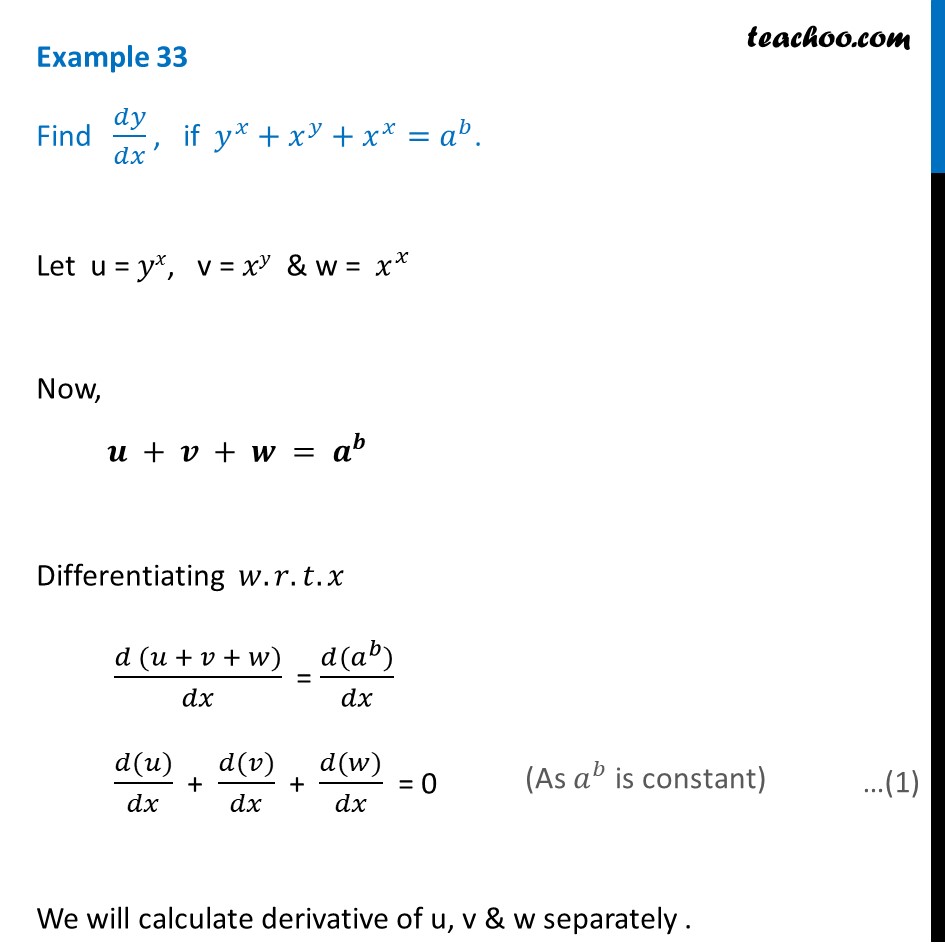



Example 33 Find Dy Dx If Y X X Y X X A B Teachoo




Differentiate The Function With Respect To X X Xcosx X 2 1 X 2 1 Mathematics Shaalaa Com
The derivative `dy/dx` has to be determined First, take the natural log of both the sides As `y = x^(ln x)` , `ln y = ln(x^(ln x))` Use the logarithmic formula `ln a^b = b*ln a`In your problem, the base y is actually a function of x, and so the rule doesn't apply Thus to properly compute the derivative, you have to rewrite y x = e x lnAnswer link mason m We can also rewrite the function from the outset to avoid fractions xy = x y Then we see that the righthand side will use the quotient rule d dx (uv) = u'v uv' Recall that differentiating anything with y will cause dy dx to spit out thanks to the chain rule Differentiating gives




Lesson 13 Derivatives Of Logarithmic And Exponential Functions




Ipe Material Notes
3 Eliminate the exponent Using the rules of logarithms, this equation can be simplified to eliminate the exponent The exponent within the logarithm function can be removed as a multiple in front of the logarithm, as follows ln y = x ln a {\displaystyle \ln y=x\ln a} 4 DifferentiateFind dy/dx xe^y=xy xey = x − y x e y = x y Differentiate both sides of the equation d dx (xey) = d dx (x−y) d d x ( x e y) = d d x ( x y) Differentiate the left side ofSteps math\dfrac{d}{dx}\left(x^2e^x\sin \left(x\right)\right)/math Apply the power rule math\left(f\cdot g\right)'=f'\cdot gf\cdot g' f=x^2,\g=e^x\sin \left
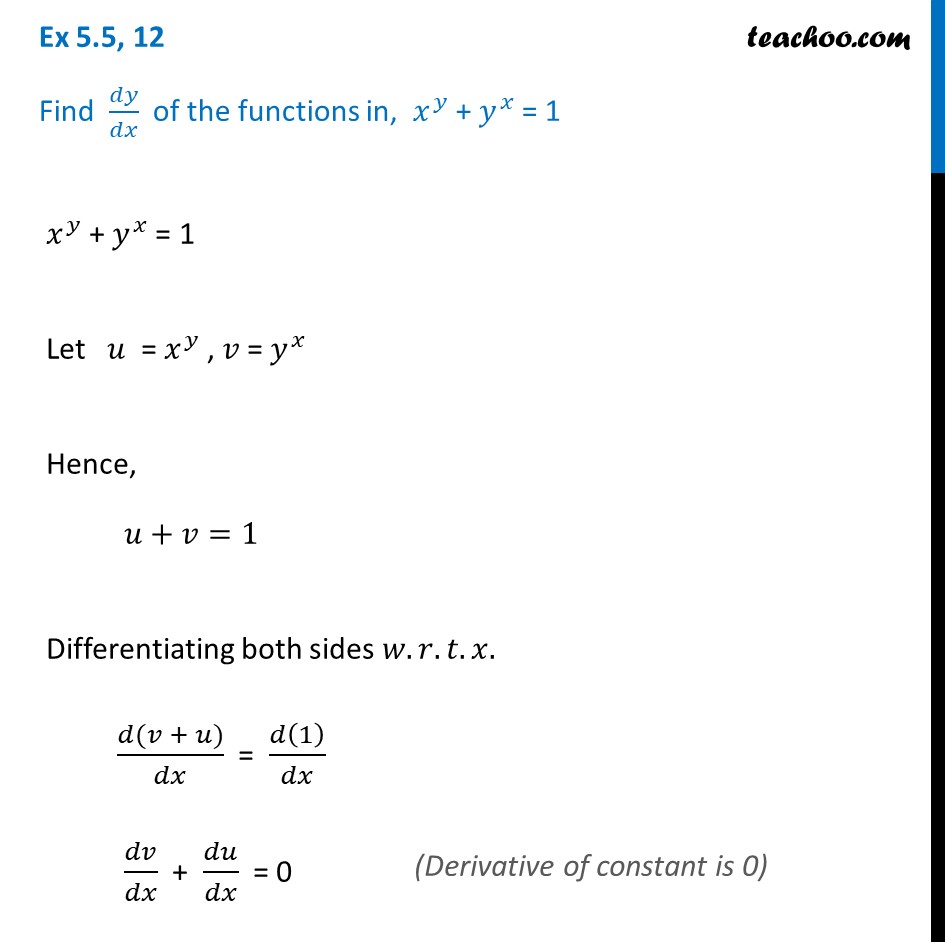



Ex 5 5 12 Find Dy Dx Xy Yx 1 Class 12 Cbse Ncert
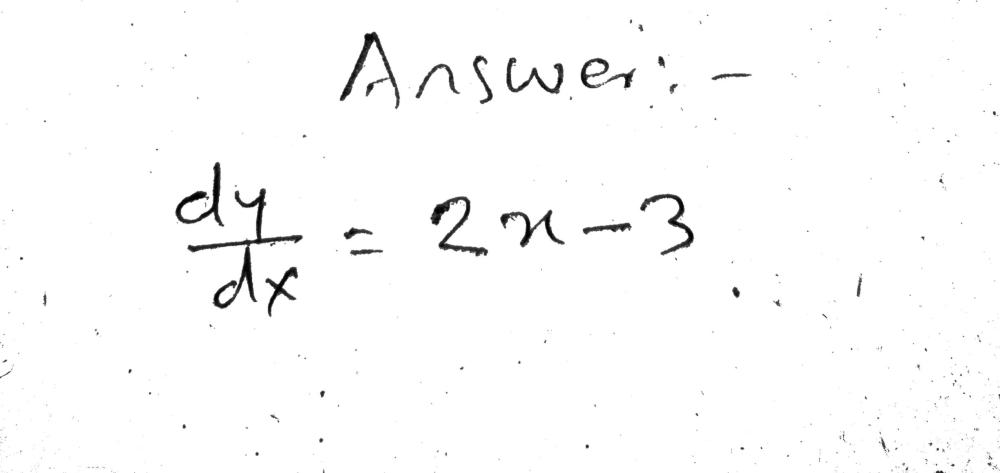



Ssscetys 5 Differentiate With Respect To X Y X Gauthmath


